The Problem of Convective Moistening

Kerry A. Emanuel

Program in Atmospheres, Oceans, and Climate

Massachusetts Institute of Technology

Cambridge, MA 02139

The problem of parameterizing cumulus convection in large-scale and mesoscale models is usually targeted toward the simulation of convective precipitation and the associated heating of the atmosphere, with a great deal of attention paid to the closure condition for the cumulus mass fluxes. By contrast, little attention has been directed to the problem of moistening and drying by ensembles of convective clouds. The degree to which convection moistens the atmosphere is strongly governed by the microphysical characteristics of clouds, which determine how much of the convective water flux is returned to the surface as precipitation and how much is used to humidify the atmosphere. Thus the problem of convective moistening hinges sensitively on a correct accounting of the microphysical processes.

That the problem of convective moistening has been paid scant attention is obvious from a quick survey of the moist popular cumulus schemes used in global and mesoscale models. In moist convective adjustment (Manabe et al., 1965), all of the condensed water is simply removed from the column without re-evaporation. There are many variants of the Kuo scheme, but they generally take an extremely simple approach to the partitioning between convection moistening and precipitation, typically representing the partitioning by a single parameter. Of the 28 journal-page description of the Arakawa-Schubert (1974) scheme, a single line in an appendix is devoted to a discussion of the microphysics of precipitation formation. More serious even than the scant attention paid to microphysics is the paucity of attempts to rigorously test against observations the fidelity of moistening produced by these schemes under controlled conditions.

Single-column models of radiative-convective equilibria indeed show great sensitivity to the way water is treated in representations of cumulus convection. Rennò et al. (1994) showed large variability in equilibrium states and climate sensitivities among various convective schemes and with variations of microphysical parameters within individual schemes.

It is straightforward to show that the correct prediction of relative humidity tendencies by a convection scheme virtually insures the correct prediction of temperature as a by-product. Consider the moist enthalpy (Emanuel, 1994), written here as

,

where is a water vapor-weighted heat capacity at constant pressure, is the latent heat of vaporization, T is the temperature and q is the specific humidity. Convection cannot change the mass-weighted vertical integral of k, since it can only convert energy from one form to another and because most of the kinetic energy is locally dissipated. Thus the correct prediction of the vertically integrated specific humidity tendency owing to moist convection necessarily entails the correct prediction of the vertically integrated temperature tendency. But in moist convecting layers, the vertical profile of temperature is strongly constrained to lie near a moist adiabat. Thus the correct prediction of the vertically integrated specific humidity tendency by convection nearly entails the correct prediction of the convective temperature tendency at each level.

In this paper I briefly describe a new convection scheme designed specifically with accurate prediction of water vapor in mind. I also describe a procedure for rigorously evaluating convection schemes using field experimental data and show some results of such an evaluation.

Details of the design of the new scheme may be found in Emanuel and Zivkovic-Rothman (1999; hereafter EZ). The basis of the new convective scheme is the representation of convection described earlier by the author (Emanuel, 1991; hereafter E91). It was recognized, in designing that scheme, that a good representation of convection should be consonant with important observed properties of cumulus clouds, including

1. the ability of deep cumulonimbi to penetrate to the level of neutral buoyancy of undiluted subcloud-layer air;
2. notwithstanding (a), the fact that the mass of a convective cloud is composed mostly of entrained air;
3. saturated downdrafts can be as strong as updrafts in nonprecipitating cumulus clouds; and
4. unsaturated downdrafts driven by evaporation of precipitation are important agents of transport and are critical for restabilizing the boundary layer.

Observations (a) and (c) decisively rule out the notion that convective clouds can be modeled as entraining plumes, as is done in several schemes, including that of Arakawa and Schubert (1974). The only way that entraining plumes can be made to conform to observations (a) and (b) together is to assume that the deepest plumes do not entrain at all; this is radically at odds with observations. And, of course, entraining plumes cannot account for observation (c) at all. The plume model cannot mimic the transports of nonprecipitating cumuli, in which updrafts and downdrafts are equally important (as there is no net heating by such clouds), except by detraining condensed water from their tops and thus concentrating the evaporative cooling there. Partially for this reason, many models artificially divide the spectrum of convective clouds into shallow and deep clouds and represent each by separate parameterizations. As the real spectrum of convective clouds is continuous, if bimodal, a unified treatment of the clouds is clearly desirable.

For these reasons, the scheme of E91 was based on the buoyancy sorting hypothesis of Raymond and Blyth (1986), which is consistent with observations (a) and (b) above and with other observed properties of real clouds (Taylor and Baker, 1991) and of high-resolution numerical simulations of shallow cumulus clouds (Carpenter et al., 1999). It assumes that mixing in clouds is highly episodic and inhomogeneous, rather than continuous as in the entraining plume model. Air that is mixed into a cloud from the environment is assumed to form a spectrum of mixtures of differing mixing fraction, which then ascend or descend to their respective levels of neutral buoyancy. In this respect, the present scheme is unchanged from that of E91.

The buoyancy sorting hypothesis by itself leaves open the issue of the rate of mixing between the plume and its environment. This is one of the great unsolved problems in cumulus convection. In laboratory plumes, experiments and dimensional analysis strongly support the contention that mixing is a simple function of the mean upward velocity of the plume. But in penetrative convection, the stable stratification of the environment evidently modifies the rate of entrainment and detrainment. The present mixing formulation is loosely fashioned after the modeling work by Bretherton and Smolarkiewicz (1989), which suggests that entrainment and detrainment rates are functions of the vertical gradients of buoyancy in clouds. The fraction of the total cloud base mass flux, M_b, that mixes with its environment at any level is here set proportional to the rate of change with altitude of the undiluted buoyancy:

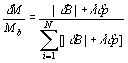(1)

Here is the rate of mixing of undiluted cloud air, M_b is the net upward mass flux through cloud base, is an entrainment parameter, B is the buoyancy of undiluted cloud air, and is the change in undiluted buoyancy over a pressure interval . As described in E91, the mixing, , can result in either entrainment or detrainment, according to the buoyancy of the mixtures. The absolute value operation in (1) reflects this fact: increasing buoyancy with height can be expected to enhance entrainment while decreasing buoyancy enhances detrainment; either increases the rate of mixing as it is defined here.

The buoyancy-sorting hypothesis (as described in detail in E91) together with the mixing hypothesis (1) together determine the net mass flux, given the net upward mass flux, M_b, of undiluted air through cloud base. The latter is determined according to the simple and elegant subcloud-layer quasi-equilibrium hypothesis (Raymond, 1995), which states that convective mass fluxes will adjust so that air within the subcloud layer remains neutrally buoyant with respect to upward displacements to just above the top of the subcloud layer. This is based on the idea that the time scale for surface fluxes and radiative cooling to destabilize the subcloud layer is relatively short. In the present implementation, we relax the cloud base upward mass flux, M_b, toward subcloud-layer quasi-equilibrium according to

, (2)

where is a fixed parameter, is the density temperature of a parcel lifted adiabatically from the subcloud layer, is the environmental density temperature, and the time step. A small damping effect is given by D. The right-hand side of (2) is evaluated at the lifted condensation level (LCL). The LCL normally occurs between model levels, so (2) is evaluated by extrapolating the parcel density temperature upward from the first level below the LCL assuming a dry adiabatic lapse rate, while the environmental density temperature is extrapolated downward from the first level above the LCL assuming a reversible moist adiabatic lapse rate. In (2), is a specified temperature deficit at the LCL, which accounts for the ability of boundary layer turbulence to overcome negative buoyancy at the LCL. In principle, should be a function of the turbulence kinetic energy at the LCL, but here we take it to be constant. The effect of (2) is to adjust M_b so that -tends to remain constant.

As mentioned in the Introduction, a primary concern in formulating a convective scheme for use in climate models is the microphysics. One is limited, on the other hand, by the fact that the clouds are parameterized and thus lack real spatial and temporal variability. Of principal concern is the fraction of condensed water that is converted to precipitation, as the remainder will contribute to moistening the environment. The two principal precipitation-forming processes in clouds are stochastic coalescence and the Bergeron-Findeisen mechanism. The efficiency with which stochastic coalescence forms precipitation is such a nonlinear function of the amount of cloud water that it is often represented as a step function of the cloud water content (Kessler, 1969). Here we adopt that philosophy by converting all cloud water in excess of a threshold content to precipitation within each sample of cloud air. Ice processes are crudely accounted for by allowing this threshold water content to be temperature dependent: While it is easy to be critical of such a simple formulation, it should be noted that it is similar to formulations used in cloud models and, more importantly, that the three convective schemes most widely used in climate models make no attempt at all to account for the nonlinearity of stochastic coalescence. No other interaction between cloud water and precipitation is accounted for.

The precipitation is added to a single, hydrostatic, unsaturated downdraft of assumed constant horizontal cross section. This downdraft transports heat and water substance, and precipitation evaporates according to a standard rate equation. Details of this formulation may be found in E91. We note here that it is necessary to assume that a specified fraction of the precipitation shaft falls through unperturbed environmental air, and that the unsaturated downdraft occupies a specified fractional area.

The scheme operates by first finding that model level, below the level of minimum moist static energy, that has the highest value of moist static energy. Convection is assumed to originate from that level. Thus, elevated convection is possible but cannot occur simultaneously with convection originating in the boundary layer.

Given the large parameter sets associated with schemes that aim toward realism, there is a strong incentive to devise rigorous observational tests of the performance of the schemes. With the notable exception of the work of Betts and Miller (1986), such tests are almost entirely absent from the literature. It is more usual for convective schemes to be subjected to semi-prognostic tests. In such tests, a single-column model containing the convective scheme is driven by forcing derived from an array of soundings. The problem with such tests is that the local time tendency, which is what one needs in the end, is usually a very small residual difference between large-scale and convective forcings. Thus a convection scheme may very well produce apparently good agreement between predicted and budget-derived convective tendencies, but at the same time the fractionally small difference between the two may nonetheless imply very large errors in the time tendencies.

A better method was advocated by E91 and Randall et al. (1996) and has been used by Sud and Walker (1993). This consists of using a single-column model to make actual predictions of temperature and humidity over a period of time long enough for these quantities to reach statistical equilibrium. But, as shown by Rennò et al. (1994), the adjustment time is of order 20 days. This time scale is set by the time necessary for a sample of air to subside through the troposphere under the influence of radiative cooling and illustrates a fundamental point about prediction of atmospheric properties in convecting regimes: adjustments of water vapor and temperature profiles depend critically on radiation, even when other processes dominate the heat and water budgets. This is because, in the clear air between clouds, the vertical velocity is always strongly constrained by the radiative cooling rate.

The prognostic, single-column test consists in integrating conservation equations for heat and moisture, with the advection terms, surfaces fluxes and radiation supplied by observations and the convection terms supplied by the convection scheme. By the abovementioned argument, these equations must be integrated over a period of at least 10-20 days for the test to be viable. For the tests described here, we use data from the Intensive Flux Array (IFA) operated in the western equatorial Pacific from 1 November 1992 to 28 February 1993 as part of the Tropical Oceans Global Atmosphere (TOGA) Coupled Ocean-Atmosphere Research Experiment (COARE). Rawinsonde soundings were taken every six hours at the IFA stations. These and other date sources were used to calculate the average temperature and specific humidity over the array as well as all the advective terms in the heat and moisture equations. The pressure velocity was calculated from horizontal winds using mass continuity with a correction applied to force the vertical velocity to vanish at 100 mb. Details of the procedure for estimating advections and vertical velocity over the IFA may be found in Lin and Johnson (1996). Surface sensible and latent heat fluxes were derived every hour from moored buoys, as described by Weller and Anderson (1996). Dry turbulent fluxes in the subcloud layer were represented by a dry adiabatic adjustment algorithm and it is assumed that dry turbulent fluxes are negligible above convective cloud base. Radiative fluxes during TOGA/COARE were observed only at the surface, by moored buoys (Weller and Anderson, 1996), and at the top of the atmosphere, by satellite (Zhang et al., 1995; Rossow and Zhang, 1995). Thus, there are no direct observations that yield estimates of the vertical structure of the radiative heating of the atmosphere. To use these in a single-column model, either an assumption must be made about the structure of the radiative heating or a radiative transfer code must be employed. We chose the former option in order to adhere most closely to the philosophy of using observations to estimate all the terms in the heat and moisture equations, except those owing to convection. While there is much confidence in radiative transfer calculations in clear-sky conditions, the strong effects of clouds can only be represented parametrically in a single-column model, introducing another source of error. Using the observed surface and TOA radiative fluxes, one must make an assumption about the vertical distribution of the radiative heating rate. Here we simply assume that the radiative heating is uniformly distributed from the surface to 100 mb. While calculated radiative heating rates in the tropics certainly show vertical structure, there is little net gradient from the surface to the tropopause. We assume that the stratosphere is in radiative equilibrium. Calculations using radiative transfer codes show that while such an assumption is warranted averaged over a diurnal cycle, net flux convergences in the middle and upper stratosphere, owing to ozone absorption, can be of order during the daytime. The effect of neglecting this will be to exaggerate the diurnal cycle of radiative heating of the troposphere.

An important check on the quality of the data is made by forming a global enthalpy conservation equation which, as mentioned before, does not need to use the convection parameterization. Using the observations of the advections, surface fluxes, and surface and TOA radiation, the integrated enthalpy equation was integrated over the entire 120-day period of operation of the IFA. At the end of the period, the predicted value of the vertically integrated enthalpy is less than the observed value by the equivalent of a 25-K temperature error integrated over the troposphere, corresponding to an average error in the net energy input of . This is a serious error and it shows that the predicted column enthalpy will have serious errors regardless of the performance of the convection scheme, unless measures are taken to correct the data. Identifying and correcting these errors is complicated and is treated extensively in EZ. Here, the IFA observations, corrected to insure global enthalpy conservation, are used to drive the single-column model evaluations.

As described in EZ, the single-column model was integrated over the entire 120 day period of operation of the IFA, and the parameters were adjusted (with the aid of the adjoint of the linear tangent of the single-column model) to minimize the root-mean-square relative humidity error, accumulated over the last 100 days and up to the 300 mb level (beyond which relative humidity observations are deemed unreliable). The results are shown here in Figure 1, which graphs the observed and predicted relative humidity, averaged over the last 100 days of the run.

The optimization succeeds in reducing the root-mean-square relative humidity error to about 15%. The large disagreement near the tropopause reflects the inability of the rawinsonde humidity element to operate at the very low temperatures found there. Observations made with special humidity sensors during CEPEX show that ice saturation at the tropopause is common.

We evaluated the optimized convection scheme by running it in the single-column model forced using an entirely independent data set. In this case, we chose to use data collected during the GARP Atlantic Tropical Experiment (GATE), conducted in the eastern tropical North Atlantic in 1974. The time evolution and all the advections of temperature and specific humidity were obtained from an archive maintained at Colorado State University. The vertical profile of radiative cooling was calculated by Cox and Griffith (1979) and represents an average over the 21 days of Phase III of GATE. We did not account for this variation of the radiative cooling profiles, nor did we attempt to correct the enthalpy budget terms as described previously for the TOGA/COARE data. Ideally, one would like a somewhat longer time series of data which has been rigorously corrected to insure global enthalpy conservation.

The results of this evaluation are shown in Figure 2 and compared to the same test run using a suite of different cumulus convection schemes. The schemes used include the untuned, older version of this scheme (Emanuel, 1991), the Betts-Miller-Janjic scheme (Janjic, 1994), and a simplified Arakawa-Schubert scheme (Pan and Wu, 1995). The last two schemes are versions currently in use in numerical weather prediction models operated by the National Centers for Environmental Prediction (NCEP). The Betts-Miller-Janjic scheme run by NCEP contains a number of significant modifications from the original Betts scheme (Betts, 1986).

The performance of the new scheme is comparable to that of the Betts-Miller-Janjic scheme, which was tuned using the GATE data, and is somewhat better than that of the other schemes tested.

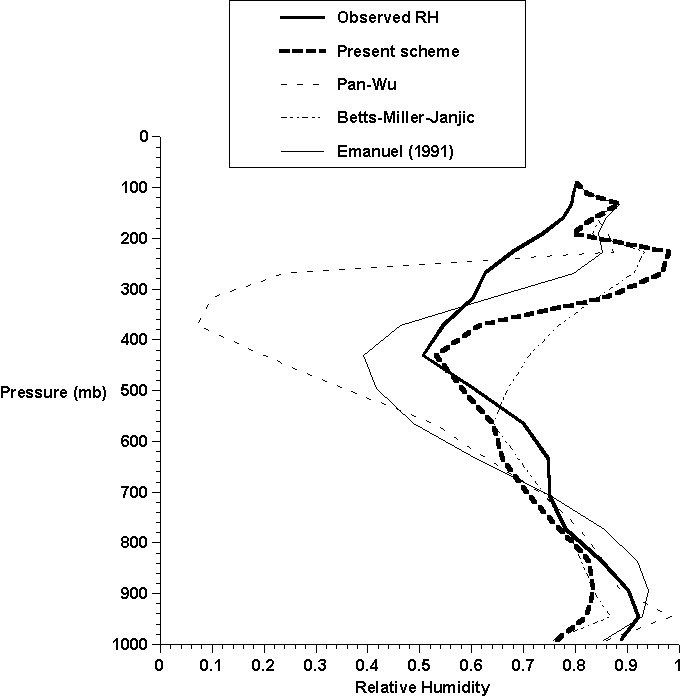
Figure 2: Comparison between observed and predicted relative humidities for the present scheme, for the original version of the scheme (Emanuel, 1991), and for the schemes of Pan-Wu (1995) and Janjic (1994). The fields are averaged over the last 8 days of GATE Phase III.

We described here a convection scheme that has been designed to yield accurate predictions of the evolution of atmospheric water vapor. To do so requires an accurate account of the important microphysical processes operating in convective clouds, but such an accounting introduces a potentially large number of adjustable parameters into the scheme. To deal with this, it is necessary to design very rigorous, off-line tests of the scheme of the type described in this paper. The parameters should be optimized using one set of field experimental data, but evaluated using an independent data set, and care must be taken to insure that the data satisfies global enthalpy conservation. Although not demonstrated in this paper (but described in EZ), a vertical grid spacing of no more than 50 mb (even in the middle troposphere) must be used for accurate prediction of humidity, otherwise the water budget may be seriously affected by numerical truncation errors.

A great many individuals and organizations contributed to this work. We would like to thank, in particular, Paul Ciesielski, Richard Johnson, and Steven Krueger for providing and assisting with the TOGA/COARE data sets, Steven Lord, Hua-Lu Pan, Zavisa Janjic, and Tom Black of NCEP for providing the convection schemes used for the comparison tests, and Joel Sloman for his assistance in preparing the manuscript. This work was supported by the U.S. Department of Energy under grant DE-FG02-91ER61220.

Arakawa, A., and W. H. Schubert, 1974: Interaction of a cumulus cloud ensemble with the large-scale environment, Part I. J. Atmos. Sci., 31, 674-701.

Betts, A. K., 1982: Saturation point analysis of moist convective overturning. J. Atmos. Sci., 39, 1484-1505.

Betts, A. K., 1986: A new convective adjustment scheme. Part I: Observational and theoretical basis. Quart. J. Roy. Meteor. Soc., 112, 677-691.

Betts, A. K., and M. J. Miller, 1986: A new convective adjustment scheme. Part II: Single column tests using GATE wave, BOMEX, ATEX and arctic air-mass data sets. Quart. J. Roy. Meteor. Soc., 112, 693-709.

Bretherton, C. S., and P. K. Smolarkiewicz, 1989: Gravity waves, compensating subsidence and detrainment around cumulus clouds. J. Atmos. Sci., 46, 740-759.

Carpenter, R. L., Jr., K. K. Droegemeier, and A. M. Blyth, 1999: Entrainment and detrainment in numerically simulated cumulus congestus clouds. Part I: General results. J. Atmos. Sci., submitted.

Cox, S. K., and K. T. Griffith, 1979: Estimates of radiative divergence during Phase III of the GARP Atlantic Tropical Experiment. Part II: Analysis of Phase III results. J. Atmos. Sci., 36, 586-601.

Emanuel, K. A., 1991: A scheme for representing cumulus convection in large-scale models. J. Atmos. Sci., 48, 2313-2335.

Janjic, Z. I., 1994: The step-mountain eta coordinate model: further developments of the convection, viscous sublayer and turbulence closure schemes. Mon. Wea. Rev., 122, 927-945.

Kuo, H.-L., 1974: Further studies of the parameterization of the influence of cumulus convection on large-scale flow. J. Atmos. Sci., 31, 1232-1240.

Lin, X., and R. H. Johnson, 1996: Kinematic and thermodynamic characteristics of the flow over the western Pacific warm pool during TOGA COARE. J. Atmos. Sci., 53, 695-715.

Manabe, S., J. Smagorinsky, and R. F. Strickler, 1965: Simulated climatology of a general circulation model with a hydrologic cycle. Mon. Wea. Rev., 93, 769-798.

Pan, H.-L., and W.-S. Wu, 1995: Implementing a mass flux convection parameterization package for the NMC Medium-Range Forecast Model. NMC Office Note No. 409, 40 pp. [Available form the National Centers for Environmental Prediction, Stop 9910, 4700 Silver Hill Road, Washington, DC 20233-9910.]

Randall, D. A., K.-M. Xu, R. J. C. Somerville, and S. Iacobellis, 1996: Single-column models and cloud ensemble models as links between observations and climate models. J. Climate, 9, 1683-1697.

Raymond, D. J., 1995: Regulation of moist convection over the west Pacific warm pool. J. Atmos. Sci., 52, 3945-3959.

Raymond, D., and A. M. Blyth, 1986: A stochastic model for nonprecipitating cumulus clouds. J. Atmos. Sci., 43, 2708-2718.

Rennò, N. O., Emanuel, K. A., and P. H. Stone, 1994: Radiative-convective model with an explicit hydrological cycle, Part I: Formulation and sensitivity to model parameters. J. Geophys. Res., 99, 14429-14441.

Rossow, W. B., and Y.-C. Zhang, 1995: Calculation of surface and top of atmosphere radiative fluxes from physical quantities based on ISCCP data sets. 2: Validation and first results. J. Geophys. Res., 100, 1167-1197.

Sud, Y. C., and G. K. Walker, 1993: A rain evaporation and downdraft parameterization to complement a cumulus updraft scheme and its evaluation using GATE data. Mon. Wea. Rev., 121, 3019-3039.

Taylor, G. R., and M. B. Baker, 1991: Entrainment and detrainment in cumulus clouds. J. Atmos. Sci., 48, 112-121.

Weller, R. A., and S. P. Anderson, 1996: Surface meteorology and air-sea fluxes in the western equatorial Pacific warm pool during TOGA coupled ocean-atmosphere response experiment. J. Climate, 9, 1959-1990.

Zhang, Y.-C., W. B. Rossow, and A. A. Lacis, 1995: Calculation of surface and top of atmosphere radiative fluxes from physical quantities based on ISCCP data sets. 1: Method and sensitivity to input data uncertainties. J. Geophys. Res., 100, 1149-1165.