Fluid Physics

8.292J/12.330J

 

Problem Set 4

 

 

1. Consider the problem of a two-dimensional (infinitely long) airplane wing traveling in the negative x direction at a speed c through an Euler fluid. In the frame of reference of the airplane, the steady flow around the wind looks like

 

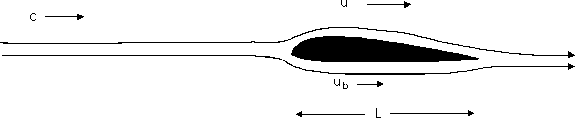
The wing has width L and the flow over its upper surface can be characterized by a speed u_t, while under the lower surface it has a speed u_b. Since the upper surface is more curved than the lower surface, u_t > u_b. The flow has a uniform density  and you may neglect gravity in this problem.

 

a.) Derive an expression for the lift on the wing, per unit length in the y direction. (Hint: Consider the pressure acting on the lower and upper surfaces of the wing.)

 

b.) In the limit that the flow speeds u_t and u_b are not very different from c, show that the lift per unit length is proportional to the circulation around the wing, with circulation defined, as usual,

 

,

 

where  is a unit vector along the wing surface.

 

c.) The oncoming flow is irrotational. What can you deduce about the lift of an airplane wing moving through an Euler fluid?

 

2. Much of the circulation of the ocean is driven by the frictional stress exerted on its surface by the wind. The essential dynamics can be understood using the vorticity equation for an incompressible fluid. Including the effect of a turbulent momentum flux, , in the vertical direction, this equation is

 

,

(1)

 

where  is the vorticity. In the ocean, the vorticity is strongly dominated by its vertical component, and the vertical component of (1) can be written, to a very good approximation, as

 

(2)

 

where  is the vertical component of velocity and  is a unit vector in the vertical direction.

 

If we approximate the flow of the ocean as steady, then

 

(3)

and using this, (2) becomes

 

(4)

 

Now we are interested in flow relative to the rotating earth. From the point of view of an observer in an inertial reference frame, the vertical component of the vorticity can be written

 

(5)

 

where  is the earth-relative velocity (i.e. the velocity we are interested in),  is the angular velocity of the earth, and  is latitude. The second term in (5) is just twice the projection of the earth's angular velocity vector onto the local vertical plane, and over most of the ocean, it is substantially larger in magnitude than the first term on the right of (5).

 

 

Next we substitute (5) into (4) and linearize the result about a state of rest (  =0), with the result that

 

(6)

 

where a is the radius of the earth, and  is the south-to-north earth-relative velocity component. Finally, for simplicity, we consider a range of latitude that is small enough that we can neglect the variability of the coefficients of (6) with latitude. Using the definitions

 

 

,

 

where  is some mean latitude, we can further approximate (6) as

 

.

(7)

 

Equation (7) says that sources of vorticity owing to stretching and to wind stress at the surface must be balanced by north-south flow, bringing in water with higher or lower rotation rates coming from higher or lower latitude. (Water at rest at the equator has no vertical component of rotation,

 

You are going to make some deductions using (7) and the incompressible mass conservation equation.

 

Consider an idealized rectangular ocean basin of dimensions  and depth H, phrased in Cartesian coordinates x, y and z. The basin is assumed to be in the Northern Hemisphere, so that both  and  are positive. Take the southern boundary of the basin to lie along , the northern boundary to lie along , the western boundary to lie along , and the eastern boundary to lie along .

 

a.) Assuming that the vertical velocity, , vanishes at both the top and bottom of the ocean, and that the wind stress also vanishes at the bottom (but not the top!), integrate (7) over the whole depth of the ocean to find an expression for the depth-averaged south-to-north velocity, .

 

b.) Integrate the mass continuity equation for an incompressible fluid through the depth of the ocean to find a differential relation between  and the depth-averaged west-to-east velocity component, .

 

c.) The wind flow over the North Atlantic ocean is dominated by an anticyclone (sometimes called the Bermuda High or the Azores High) which is associated with clockwise flow. Thus the curl of the wind stress on the ocean is almost everywhere negative, reaching a maximum amplitude in middle latitudes. For our simple ocean model, we are going to idealize this wind stress as

 

 

where  is the wind stress at the ocean surface and  is the amplitude of the wind stress curl.

 

Using the result of (a) above, find an expression for . Now using the result of (b) above, find an expression for . Note that the boundary condition on  is that it must vanish at both the eastern and western boundaries. You will not be able to satisfy both of these conditions. So find two solutions: one that satisfies  =0 on the eastern boundary, and one that satisfies  =0 on the western boundary.

 

d.) The reason that both boundary conditions on  cannot be satisfied is that there exists at either the western or the eastern boundary a thin boundary layer in which all of the "return flow" is concentrated and in which some of the approximations we used break down. The most important approximation is the neglect of the friction of the ocean flowing along the side boundaries. One effect of this friction is to change the vorticity of the fluid. Note that the wind stress in our problem provides a negative definite basin-averaged vorticity tendency. In the steady state, this will have to be balanced by a source. Considering qualitatively how the side boundary friction might affect vorticity, at which boundary (east or west) do you think the return flow is concentrated?  Explain your reasoning, and sketch what the flow might look like (including the boundary layer).

 

Extra Credit:  The effect of side boundary friction can be modeled by adding a term

 

 

to the right-hand side of (2), where  is a coefficient which you may assume is constant. This term is negligible in the interior, where the solution we obtained before is valid. But it dominates the right side of (2) in the thin boundary layer. By obtaining a solution for the thin boundary layer and matching this to the interior solution at the outer edge of the boundary layer, find a solution that satisfies both boundary conditions on  and also satisfies  on the continental side of the boundary layer.